- Messages
- 6,337
He’s going to strain his back from moving the goalpost now.Nate wanted a good PA poll. Delivered.
Follow along with the video below to see how to install our site as a web app on your home screen.
Note: This feature may not be available in some browsers.
He’s going to strain his back from moving the goalpost now.Nate wanted a good PA poll. Delivered.
My suspicion is if she loses PA, the reasons will not be sufficiently state specific. And frankly the math gets very difficult from there.1. It depends on why she loses PA. If it's a state specific thing, then arguably she might be able to offset that loss with NC or GA. There's no inherent reason why she can't win either of those states. They have a bigger R partisan lean than PA, but again, if the PA thing is state-specific (i.e. fracking), then it's not game over.
2. If she wins PA, she's very likely to take MI and WI and that's pretty much all she needs.
3. And we get another idiocy of the electoral college. AZ + NV is enough to make up the loss of MI. It's not enough to make up the loss of PA. Does that make sense? I mean, I guess PA has more people than MI but it's not really about the population. It's about the arithmetic. It just so happens that the 4 EVs make a difference this go round, primarily because the Dems are winning VA instead of NC and because Utah just barely squeezed ahead of MN for the last EV.
And all of this, even though the vote margin would probably be the same in Michigan as Pennsylvania -- and proportionally bigger in MI. So it's better for Kamala to do better in PA than MI, even if she does worse in the two put together. It is so frustrating.
4. I still can't believe that there is no appetite in half the country to get rid of the EC. I get it -- ideology. But there's no reason that the GOP can't win in a non-EC world. They might need to tweak their policies a bit, and make more of an effort in CA, but it's doable. Meanwhile, the vast majority of the citizens in this country actually have no say at all about the next president, because only PA, MI, WI, NC and AZ matter (and not all of them do).
Every election boils down to four or five swing states. A constitutional amendment can pass with 38 states approving. It would undoubtedly be better for the 45 non-swing states to get rid of the EC and make their votes matter. And yet. And yet.
What don you think of MAGAs comparing the same day polls in ‘16 and ‘20 polls to extrapolate what will happen ‘24?I knew that bump was coming.
YeaThis is an election like none other we’ve ever seen. I don’t believe that this historical data is very pertinent.
When you've lost RCP...
With the Harris campaign putting money into NC and FL (and gaining ground there), you force Trump to do the same. And given the respective sizes of the war chests (I think that Harris had almost 2x as much money), that bleeding of Trump's money in NC/FL results in less money in other swing states, especially PA.My suspicion is if she loses PA, the reasons will not be sufficiently state specific. And frankly the math gets very difficult from there.
NC going blue is, to me, a bit of a pipe dream. It took 8 years of Bush, an economic collapse and a generational talent in Obama to flip NC blue. And he managed that by 0.3%. And went 1 for 2 (losing the state to Romney in 2012). With Kamala trailing slightly in polling…it’s hard to see her winning here especially when I think she needs to stay so locked in on Michigan, Wisconsin and PA.
GA did surprise me last election though. But if I was her, I’d spend all that political capital and ground game in PA and Wisconsin. I think NC is likely a fools errand unless she was reliably outpolling Trump here.
The EC isn’t going away anytime soon so Democrats have to consider how to fine tune their messaging to make the Democratic coalition stronger in the Electoral College.
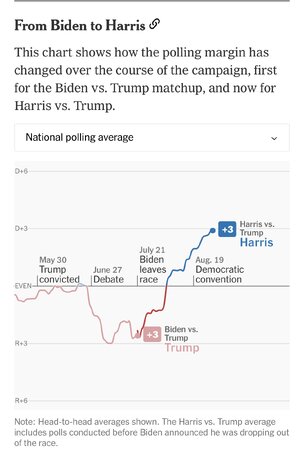
Still would be folks not captured previously plus momentum and enthusiasmThe key question is how much of that is catching up from registrations that were flagging for months under Biden versus an indication of a year-over-election-year surge in these sorts of registrations?
